Symmetric Matrix In Complex
U Tu u TAu u TAu ATu Tu since BvT vTBT. A 3 5 5 8.
What Is A Skew Symmetric Matrix Quora
Central normal complex random vectors that are circularly symmetric are of particular interest because they are fully specified by the covariance matrix.
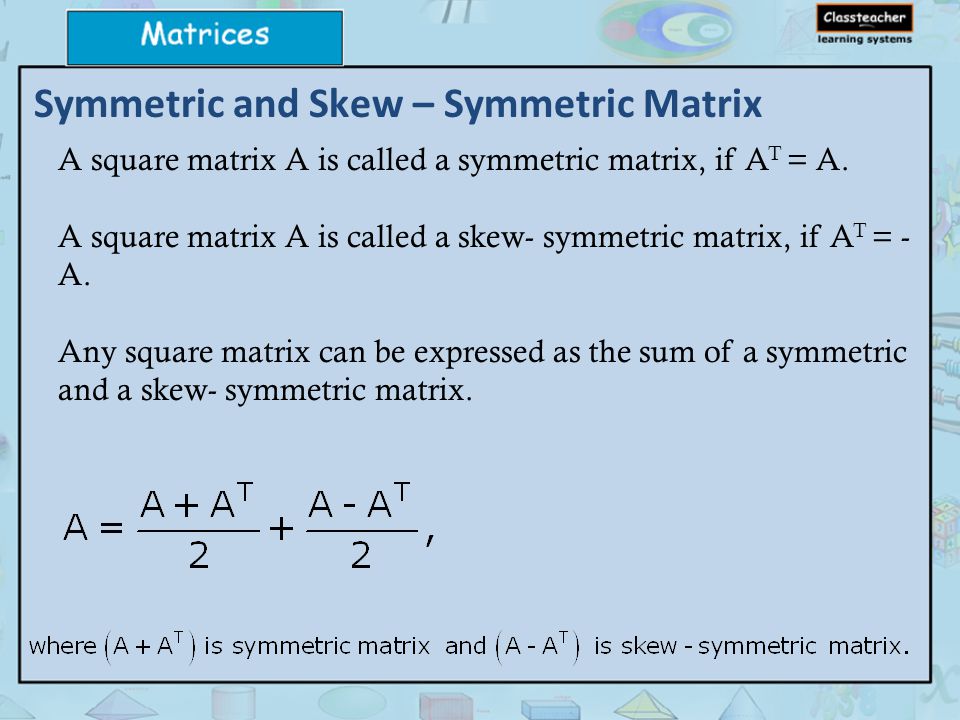
Symmetric matrix in complex. If alpha is a complex number then clearly you have a complex eigenvector. A 1 23 i 8 23i 4 6 7i 8 67 i 5. Suppose A is symmetric and Ax λx.
Such complex symmetric matrices arise naturally in the study of damped vibrations of linear systems. A 6 84 i 84i 9. To be more specific a complex symmetric matrix is a square matrix T with complex entries such that T Tt the superscript t denotes the transpose oper-ation and two matrices AB M nC are unitarily equivalent if there exists a unitary U M nC such that A UBU.
Then S-1 AS Ʌ becomes special - it is Q-1 AQ or A Q Q-1 Now we can state one of the great theorms of Linear algebra. There is such a thing as a complex-symmetric matrix aij aji - a complex symmetric matrix need not have real diagonal entries. As mentioned above hermitian matrices are the complex analogue of symmetric matrices.
K is complex symmetric Simplest case. A 2 1 1 4. Indeed if vabi is an eigenvector with eigenvalue lambda then Avlambda v and vneq 0.
It is well known that a real symmetric matrix can be diagonalised by an orthogonal transformation. 2 I Now we pre-multiply 1 with u T to obtain. 1 I Taking complex conjugates of both sides of 1 we obtain.
A 0 2 4 2 7 5 4 5 8. With the idea of skew symmetry for a matrix now couched in terms of the inner product it is easy to see that eigenvectors with different eigenvalues must be orthogonal as well as the fact that. Real eigenvalues Why are the eigenvalues of a symmetric matrix real.
But with respect to its singular vectors a symmetric matrix is special as has been known for a long time 151410. This implies that no. Such complex symmetric matrices arise naturally in the study of damped vibrations of linear systems.
But if A is a real symmetric matrix AAt then its eigenvalues are real and you can always pick the corresponding eigenvectors with real entries. The circularly-symmetric central complex normal distribution corresponds to the case of zero mean and zero relation matrix ie. A 2i 34 i 34 i 87i.
For the complex symmetric n X n matrix A there exists a unitary matrix Q such that A QXQT 11 where If diag al. A u u ie Au u. For a symmetric matrix Atext we had mathbfxdotp AmathbfyAmathbfxdotp mathbfytext.
It is shown in this paper that a complex symmetric matrix can be diagonalised by a complex orthogonal. An n n complex matrix is Hermitian if A A. For any sesquilinear pairing P on C n there is a unique n n matrix A P such that P v w w T A P v w A P v Moreover if P is conjugate-symmetric then A P is Hermitian.
In addition any matrix of the form QΛQT will be symmetric. This proves that complex eigenvalues of real valued matrices come in conjugate pairs. This statement is not true in general for a symmetric matrix of complex elements.
In other words there is a complex orthogonal rather than unitary matrix of eigenvectors. Its orthonormal eigenvectors are in the orthogonal matrix Q and its eigenvalues are in where diag λ. A real matrix is symmetric if we now say that a complex matrix is skew symmetric if In view of this equality the defining property of symmetric and skew-symmetric matrices becomes.
In fact any real or complex n X n matrix is similar to a complex symmetric matrix see eg. These are exactly the possibly complex-valued symmetric matrix. A real symmetric matrix can be factored into AQQT.
Into an orthogonal matrix Q with QT Q I and QT Q-1. It is shown in this paper that a complex symmetric matrix can be diagonalised by a complex orthogonal transformation when and only when each eigenspace of the matrix has an orthonormal basis. μ 0 displaystyle mu 0 and C 0 displaystyle C0.
Our primary motivation stems from the emerging theory of complex symmetric. Recall that a key property of a symmetric matrix is its symmetry with respect to the dot product. Several remarks are in order.
Then we have pcC rcC NCC. Let C be a complex-valued symmet- ric matrix C C. We state our conclusion as LEMMA 21 eg.
Then we can conjugate to get Ax λx. K K0 iD Complex Symmetric Matrices p. Eigenvalues of a symmetric real matrix are real I Let 2C be an eigenvalue of a symmetric A 2Rn n and let u 2Cn be a corresponding eigenvector.
If the entries of A are real this becomes Ax λx. A symmetric matrix of complex elements. As the examples show the set of all real symmetric matrices is included.
Here are a few examples. At any rate a complex symmetric matrix M is diagonalizable if and only if its eigenvector matrix A can be chosen so that A T M A D and A T A I where D is the diagonal matrix of eigenvalues.

Symmetric And Skew Symmetric Ppt Video Online Download

Derivative Of A Rotation Matrix Robot Academy

Mathematics Symmetric Skew Symmetric And Orthogonal Matrix Youtube

What Is A Skew Symmetric Matrix Quora

Linear Algebra 101 Part 7 Eigendecomposition When Symmetric By Sho Nakagome Sho Jp Medium
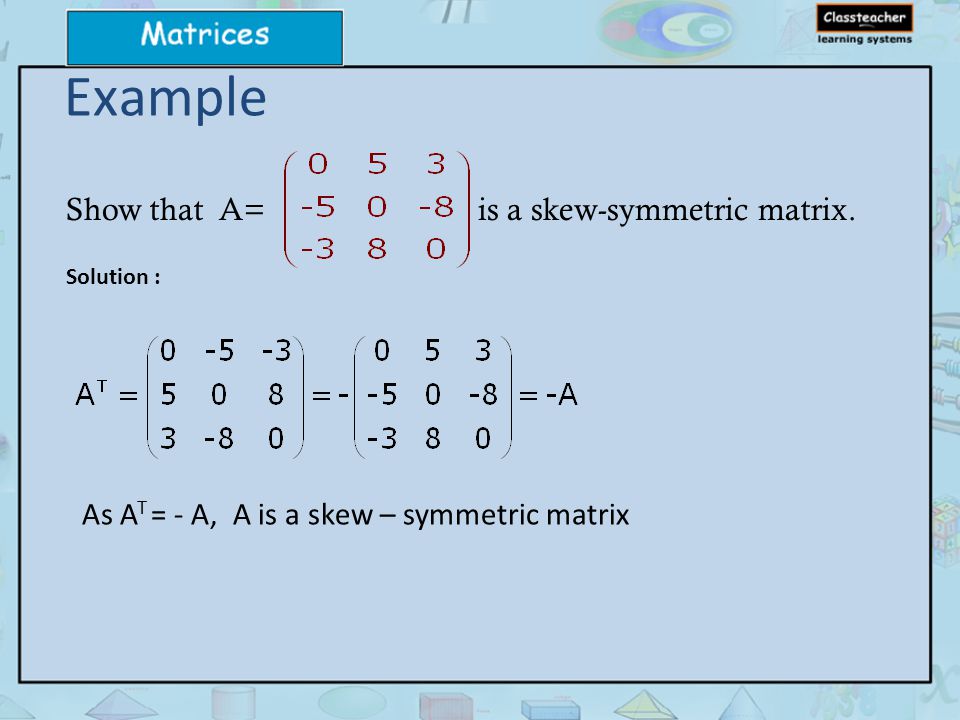
Symmetric And Skew Symmetric Ppt Video Online Download
How To Check If A Matrix Is Positive Definite Quora

Skew Symmetric Matrices Lesson Robot Academy

What Is Determinant Of Skew Symmetric Matrix
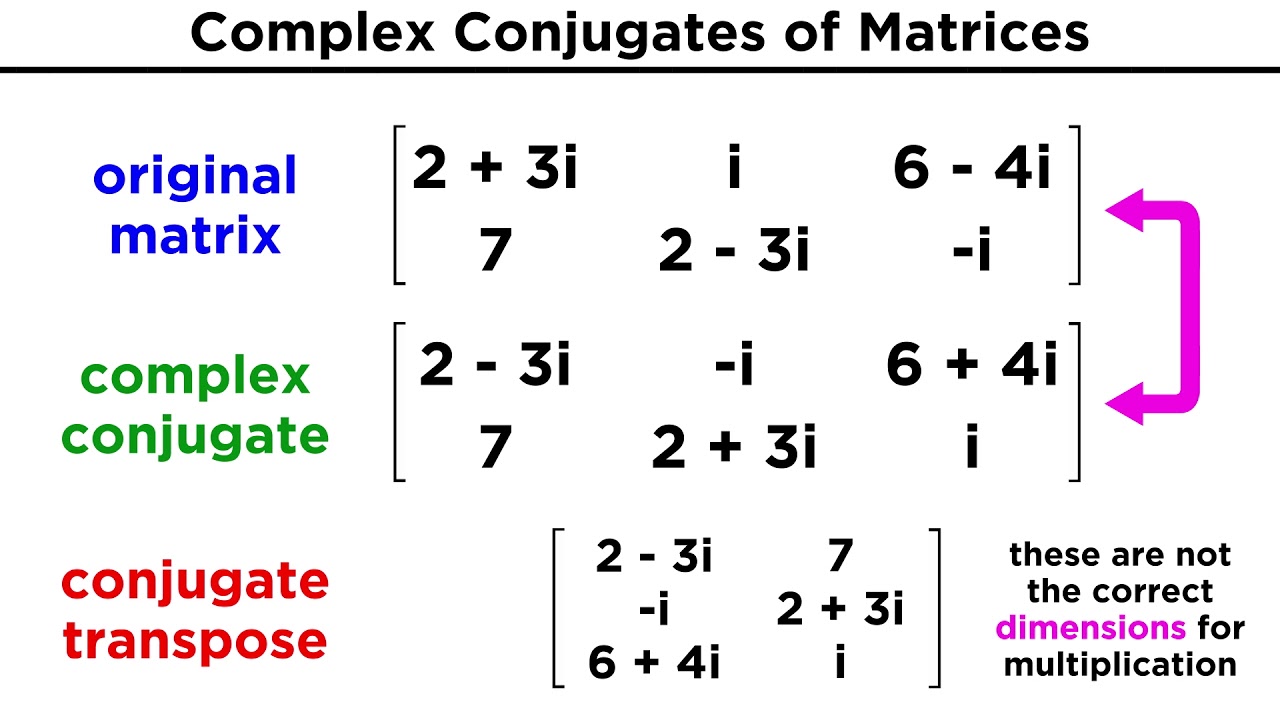
Complex Hermitian And Unitary Matrices Youtube

What Form Does A 3 3 Matrix Have If It Is Symmetric As Well As Skew Symmetric Quora

Skew Symmetric Matrices Lesson Robot Academy

What Form Does A 3 3 Matrix Have If It Is Symmetric As Well As Skew Symmetric Quora

Skew Symmetric Matrix Don T Memorise Youtube
What Is A Skew Symmetric Matrix Quora

Symmetric Matrices Eigenvalues Eigenvectors Youtube

Skew Symmetric Matrix An Overview Sciencedirect Topics

