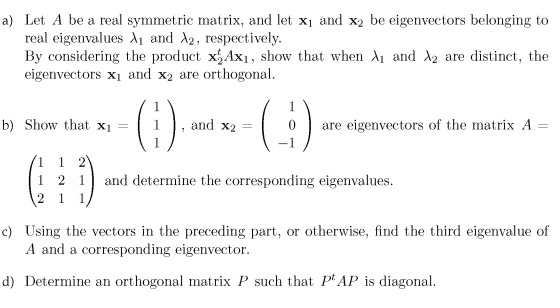
Product Of Symmetric Matrices Eigenvectors
Now we need to get the matrix into reduced echelon form. Let.
Karl Stratos Research Eigentutorial
Given two eigenvectors of a symmetric matrix with different eigenval.
Product of symmetric matrices eigenvectors. Since λ 1 λ 2 0 x y 0. Because Pis symmetric and orthogonal Pis its own inverse so Pe 1 x. THE EIGENVALUES OF RANDOM SYMMETRIC MATRICES 237 The expectation of a particular product aioi.
A Square Root Matrix of a Symmetric Matrix with Non-Negative Eigenvalues Let A be an ntimes n real symmetric matrix whose eigenvalues are all non-negative real numbers. This can be reduced to This is in equation form is which can be rewritten as. Let A and B be two real symmetric matrices one of which is positive definite.
Because A is symmetric we. For any real matrix A and any vectors x and y we have. The product of two symmetric matrices is usually not symmetric.
Then we can conjugate to get Ax λx. The only eigenvalues of a projection matrix are 0 and 1. We can also write A Xd i1 iu iu T i.
Eigenvectors of symmetric matrices fact. But the left side is 0 because of the symmetry. A u u ie Au u.
P is singularso D 0 is an eigenvalue. V n 3 7 7 5 Xn i1 u iv i. 11 Positive semi-de nite matrices.
I think you can get bounds on the modulus of the eigenvalues of the product. Use the fact that a real symmetric matrix is diagonalizable by a real orthogonal matrix. W v w v.
Every generalized eigenvector of a normal matrix is an ordinary eigenvector. Now assume that A is symmetric and x and y are eigenvectors of A corresponding to distinct eigenvalues λ and μ. There are very short 1 or 2 line proofs based on considering scalars xAy where x and y are column vectors and prime is transpose that real symmetric matrices have real eigenvalues and that the eigenspaces corresponding to distinct eigenvalues are orthogonal.
C n C n C v w v w be a sesquilinear conjugate-symmetric pairing on C n represented with respect to the standard basis by the Hermitian matrix A. A slightly more precise version of the question would ask why a symmetric matrix has orthogonal eigenspaces because there is a difference here between necessity and possibility. Then is positive-definite ie is a Hermitian inner product iff all of the eigenvalues of A are positive.
Dgis an orthonormal basis consisting of eigenvectors of A and i is the eigenvalue corresponding to u i. Therefore λ μ x y 0. 1 The eigenvalues are uniquely determined by A up to reordering.
First recall that the dot product of two column vectors u and v in Rn can be written as a row by column product uv utv u1 u2u n 2 6 6 4 v1 v2. Normal Hermitian and real-symmetric matrices have several useful properties. By induction we can choose an orthonormal basis in consisting of eigenvectors of.
Matrices and most important symmetric matrices. Since it follows that is a symmetric matrix. There is a set of orthonormal eigenvectors of A ie q1qn st.
Aqi λiqi qiTqj δij in matrix form. It follows that the matrix PTAP which is a similarity transformation of A satis es PTAPe 1 PTAx PTx Px e 1. Eru_riul oz-2 Kk-2P2 since Efarft3 iljJ andhence lEorl ozp-zKk-2p2nn- t.
Show that there is an n times n real matrix B such that B2A. Real eigenvalues Why are the eigenvalues of a symmetric matrix real. 2 I Now we pre-multiply 1 with u T to obtain.
Yes eigenvectors belonging to distinct eigenvalues of symmetric positive matrix are orthogonal and your solution is correct. Then it is easy to see that the product A B or B A which has the same eigenvalues is similar to a symmetric matrix so has real eigenvalues. We need to take the dot product and set it equal to zero and pick a value for and.
It follows that is an orthonormal basis for consisting of eigenvectors of. That is e 1 is an eigenvector of PTAP with eigenvalue and therefore PTAP has the block structure PTAP vT 0 B. Take the vectors of eigenvalues of A and of B sorted in decreasing order and let their componentwise product be a b.
Finally let for. Suppose A is symmetric and Ax λx. Now we need to substitute into or matrix in order to find the eigenvectors.
λ x y λ x y A x y x A T y x A y x μ y μ x y. It is always the case for a symmetric matrix by the following reasoning. All have special s and xs.
To verify this point compute It follows that where is a symmetric matrix. In vector form it looks like. P is symmetric so its eigenvectors 11 and 1.
A x y x A T y. Q1AQ QTAQ Λ hence we can express A as A QΛQT Xn i1 λiqiq T i in particular qi are both left and right eigenvectors. Aiu_u CÍ be estimated lEaror.
This proves that complex eigenvalues of real valued matrices come in conjugate pairs Now transpose to get xT AT xTλ. Each column of P D5 55 5 adds to 1so D 1 is an eigenvalue. 1 I Taking complex conjugates of both sides of 1 we obtain.
Eigenvalues of a symmetric real matrix are real I Let 2C be an eigenvalue of a symmetric A 2Rn n and let u 2Cn be a corresponding eigenvector. A x y A x y x A T y x A y x A y. Somehow I think it can also be shown further that all real symmetric matrices have their own orthonormal basis of eigenvectors.
U Tu u TAu u TAu ATu Tu since BvT vTBT. In addition any matrix of the form QΛQT will be symmetric. The eigenvectors for D 0.
If the entries of A are real this becomes Ax λx. There is an orthogonal Q st. When applied to column vectors the adjoint can be used to define the canonical inner product on C n.
The perpendicularity of the eigenvectors is no accident.
Eigenvectors Of Symmetric Matrices Are Orthogonal Youtube
Chapter 7 Symmetric Matrices And Quadratic Forms Flashcards Quizlet
Http Www Cse Iitm Ac In Vplab Courses Larp 2018 Chap5 Evv Larp Pdf
A Let A Be A Real Symmetric Matrix And Let Xi And Chegg Com
Linear Algebra Example Problems Eigenvalue Computation 3 Youtube
How To Find The Third Eigenvector Of A 3x3 Matrix Given The Other Two Eigenvectors Mathematics Stack Exchange
Eigenvalues And Eigenvectors Of Symmetric Matrices Linear Algebra
Linear Algebra 101 Part 7 Eigendecomposition When Symmetric By Sho Nakagome Sho Jp Medium
Eigenvalue Feature 4 The Trace Youtube
5 Symmetric Matrices We Want To Show That Every Real Chegg Com
Proof For Why Symmetric Matrices Are Only Orthogonally Diagonalizable Mathematics Stack Exchange
The Number Of Positive Eigenvalues Of A Non Singular Symmetric Matrix Is Equal To The Number Of Positive Pivots Strang Mathematics Stack Exchange
Eigenvalues And Eigenvectors Of Symmetric Matrices Linear Algebra
Symmetric Matrix Decomposition With Orthonormal Basis Of Non Eigenvectors Mathematics Stack Exchange
Eigenvalues Eigenvectors 7 1 Eigenvalues Eigenvectors N N
Proof For Why Symmetric Matrices Are Only Orthogonally Diagonalizable Mathematics Stack Exchange
Eigenvalues Of Real Skew Symmetric Matrix Are Zero Or Purely Imaginary And The Rank Is Even Problems In Mathematics
Symmetric Matrices Eigenvalues Eigenvectors Youtube
What Are The General Form Of Eigenvalues Of An Orthogonal Matrix Mathematics Stack Exchange
